Extra credit is available at the end of this page. Please respond before 9 AM, Monday, March 26^th, 2001.

The rainbow is created by refraction and reflection of sunlight by water droplets. The geometry is more complicated than that of a prism, but nothing we can't handle. By considering how a few beams of light interact with an idealized spherical raindrop, we will determine a few basic facts about rainbows that you can go out and see for yourself. These are facts that you probably have already seen, but may not have really noticed.

The following discussion all refers to the diagram below. Here are a few important geometerical facts:

Lines ABC, DEF, and GHI are parallel.

The dashed lines R1, R2, and R3 are radial, and thus are perpendicular to the surface of the drop at B, E, and H.

We consider a single ray of sunlight; it enters the picture at point A and is refracted into the drop at point B. The ray hits the back of the drop at point E and is reflected to point H. There, it is refracted out of the drop and leaves the picture at point J. The ray is now traveling down towards earth at angle delta.
In order to figure out more about the rainbow produced by this beam and others like it, we will follow these steps.

1. Do some geometry and get a relationship between the incident and refracted angles (theta i and theta r) and the angle of deflection, delta.
2. Use Snell's law to get theta r in terms of theta i and the index of refraction, n. This will give us delta as a function of theta i and n.
3. We will analyze the function, and notice that for any n, delta has a maximum at some theta i.
4. At this point, we will take dispersion into account. That is, we will we will include the dependence of n on wavelength. Considering the extremes of the visible spectrum
   (n_red = 1.333 and n_violet = 1.346) we will find the maximum deflection angle, delta, for each color.
5. Finally, we will put all of the pieces together; we will imagine ourselves standing in the outside with raindrops in front of us and the sun over our shoulders. We will envision the rainbow itself.

First, notice that the triangles OBE and OEH are congruent (identical), isoceles triangles. We know this because angles BEO and OEH are the same (theta incident = theta reflected), and because line segments OE, OB and OH are all the same length. This means that angles OBE, OEB, OEH, and OHE are all the same.

To get an expression for delta, we start at B and keep track of the angle of the ray with respect to the horizontal at each point:

• At B, the ray turns Clockwise (CW) by an amount theta i - theta r
• At E, the ray turns CW by 180 - 2*(theta r).
• At H, the ray turns CW by another theta i - theta r.
• Adding these up, the ray turns by 2*(theta i)-4*(theta r)+180.
• This sum equals angle GHJ the total deflection. Which is 180-delta

Now, use Snell's law, n_asin(theta a)=n_bsin(theta b). In this case, n_a=1 theta a=theta i and theta b=theta r. so sin(theta r)=sin(theta i)/n_water. We get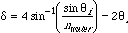

Now, let's make a quick table of values of theta i, theta r, and delta.

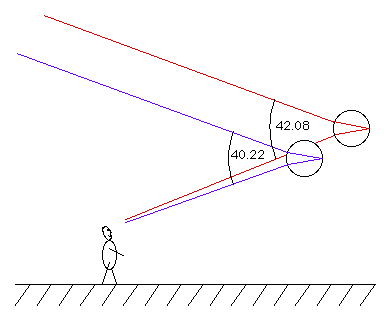
Using the expression obtained (an extra credit problem!) we find that for n=n_red delta is a maximum for theta i=59.41 degrees. For n=n_violet=1.346, delta is a maximum for theta i=58.66 degrees. Plugging these values back into the equation for delta, we find that

the maximum delta is 42.08 degrees for red light, and 40.22 degrees for violet light.

This completes step 4.

Now for step 5, the conclusion.

Imagine yourself standing with the sun at your back, exactly 20 degrees above the horizon. You are looking up at a bunch of raindrops at various angles above the horizon in front of you. For red light, the maximum delta is 42.08, so looking 42.08-20 = 22.08 degrees above the horizon, you can see only red refracted light. As you look lower down, there will still be red light, but new colors will be added one at a time: first orange, then yellow, green, etc. As each color is added, your eye perceives the series as a series of colors. When you are looking at an angle of 40.22-20 = 20.22 degrees above the horizon, the violet light will be added. The whole process takes 22.08-20.22 = 1.86 degrees. This would be the angular width of the rainbow if the sun were really a single point in the sky. Actually the sun is about 1/2 degree wide itself, so the rainbow is about 2.36 degrees wide.

A few notable features of this analysis of the rainbow:

• Outside the bow, no refracted light reaches the eye, whereas inside the bow, all colors can reach the eye. Thus, the sky is darker outside the bow than it is inside.
• The color scheme: red outside, violet inside
• The angular size of the whole bow is about 41 degrees.

You can get a lot more information about this subject on the internet. Here are a few search engines

1. Alta Vista

2. Google

3. Northern Light

4. Ask Jeeves

5. Infoseek

And here are a few good links to get you started.

1.

2.

3.

4.

This site is made possible by funding from the National Science Foundation (DUE-9981111).