What is ?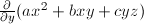

1. 0
2. 2ax+b+c
3. bx+cz
4. by+cy
5. b+c

---

Consider a 2d box which is infinite in one dimension and finite in the other. This is a reasonable model of quantum wells constructed by growing thin layers of different materials on a surface. What would you expect the energy levels to be like?

1. They would be discrete, but more spread out than in a square box.
2. They would be discrete, but closer together than in a square box.
3. Any energy would be allowed.
4. They would be the same as those in a square box.
5. It depends on the depth and width of the box.

---

How would you expect the energy levels in a cube to compare with those in a square box if both had sides of the same length?

1. They energies would be the same.
2. The cube ground state energy would be higher.
3. The cube ground state would have a higher degeneracy.
4. The degeneracies would be the same.
5. It's impossible to tell.

---

Consider the (2,1) state in a square box. What is the probability density of finding an electron in the center of the box?

1. maximum
2. zero
3. positive, but less than the maximum
4. negative, but more than the minimum
5. It's impossible to tell.

---

Which of the following potential energies will result in a 3d Schrödinger equation which is separable into radial and angular parts?

1. U=ar^1/2 + e^-r
2. U = a/r cos(θ)
3. U = r² + 2r
4. U = x/r + y/r
5. U = a (constant)

---

The solutions to the θ and φ equations in the 3d radial Schrödinger equation will depend on the precise form of the central potential.

1. True
2. False

---

Two wave functions with different values of the absolute value of their angular momentum quantum number m but with the same value for the radial quantum number n will have the same radial shape.

1. True
2. False

---

Which radial wavefunction would you expect to have the most nodes? (A node is a place where the wave function is equal to 0.)

1. n=1
2. n=2
3. n=3
4. n=4
5. n=5

---

How would the mean free path of hydrogen atoms in the n=3 state compare with the mean free path of hydrogen atoms in their ground state (n=1)? Assume the temperature and density are the same in both cases?

1. same
2. 9 times as big
3. 9 times smaller
4. 81 times as big
5. 81 times smaller

---

Since the potential energy is spherically symmetric, how do we know which direction to take as the z direction?

1. We don't. It's arbitrary.
2. It is determined by the magnetic field of the nucleus.
3. It depends on the spin of the electron.
4. It depends on the orientation of the atom.
5. It's determined by the right hand rule. Use your fingers to follow the orbit of the electron and your thumb will point in the z direction.

---

50. Which of the following are possible states in a hydrogen atom?

1. 2p, m=0
2. 1p, m=-1
3. 3d, m=2
4. 4s, m=-1
5. 2s, m=1

---
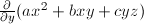